Lenses
Introduction
The heart of every camera is the lens. In recent years, amidst discussions about digital sensors, megapixel hype, etc., it has somewhat receded into the background. Nevertheless, without a good lens, one cannot create at least technically "good" shots. Typical technical parameters include sharpness, contrast, color errors (chromatic aberration), and geometric imaging performance (barrel and pincushion distortions). These parameters are naturally interdependent; optimizing one may degrade another. For example, geometric distortion may not be as important for a landscape photographer as it is for an architectural photographer, indicating that a lens may be better suited for one use case than another. But these are already details.
Today, anyone capturing images with a digital camera will likely edit them on their computer afterward; at least, that's what most dedicated photographers do. During such editing steps, various lens parameters can be corrected retroactively, often resulting in a negligible loss of quality. Chromatic aberration (color errors), vignetting (darkening of the image towards the edges), and geometric distortions are very effectively correctable afterward. All of this can be incorporated into a profile during editing, specifically calibrated for a lens at different apertures and possibly even different distances. Some cameras, such as the M8, can even compensate for vignetting in-camera, while Canon does this only in jpg format during capture. However, the article on Lens Coding demonstrates that retroactive editing with a profile is a superior method. Unfortunately, the parameters that are easily correctable are often less critical for an image. For instance, sharpness cannot be restored retroactively. While it's possible to sharpen photos afterward, a photo focused on the wrong point cannot be saved in this regard.
Here, I want to conduct a very practical comparison by examining excerpts from different shots. This has the advantage of representing a real-world scenario (unlike a pure measurement of MTF, for example), but it also has the disadvantage of covering only one case. From a single image, it's hard to determine, for instance, whether the lens reacts negatively to side sunlight (flare) or whether it performs similarly well at close and distant distances. However, as we will see, some remarkable differences between lenses can be highlighted based on a single shot.
I don't want to limit myself to just one camera system but rather introduce two systems into the comparison. Is this legitimate? Can one compare two different systems with different lenses? Absolutely! As a user, I'm primarily interested in the result. Here, I will compare a Leica M system (based on the M8) and a Canon system (based on a Canon 5DMK2) and their respective lenses. Both cameras have approximately the same pixel density; the M8 has the same pixel density as the M9, so the results would be very similar with the M9. The table below assumes that the pixels are densely packed, but in reality, there is a small gap between the pixels. Although this does not affect pixel density, the pixel size will be slightly smaller in reality. The pixel length of the 5DMK2 is less than 10% smaller than that of the M8; I have neglected this difference in the following. It is also difficult to emphasize the difference because, in image comparisons, one must map a sensor pixel to a screen pixel, as otherwise, scaling effects are factored in, inevitably altering the image's sharpness.
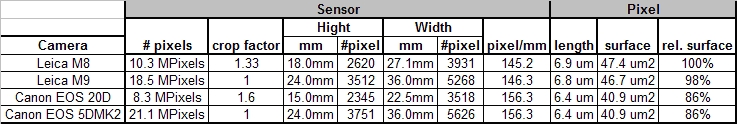
However, the M8 has a sensor that is slightly smaller than the full-frame format. The sensor has a crop factor of 1.33, meaning its side lengths are 1.33 times shorter than those of the full-frame 5DMK2. I have taken this into account in the excerpts. When I refer to the edge, I mean the outer edge of the M8; for the 5DMK2, it is not quite at the edge. However, since I use lenses for both systems designed for the full frame, this does not matter. The only disadvantage is that I cannot compare the resolution at the outermost edge of the full frame. And since an equal number of pixels are always cut out, the crop factor can be ignored. The comparison always refers to a section of n by m pixels, and this is the same size for both due to the similar pixel density. Thus, one can directly compare aperture values and disregard the equivalent aperture that one would have to consider when working with the crop factor. The crop factor and equivalent aperture only come into play when enlarging different images differently, for example, enlarging an M8 image to the same format, such as A4, as a 5DMK2 image.
To put the 100% excerpts shown below into perspective, I calculated the size that an entire image would require on the screen or how large it would become when making a print using the optimal resolution. I assumed 72lpi resolution for a screen and 240dpi for a print; the latter was determined in various tests as the minimum resolution for a print where no loss of quality is noticeable. In other words, there is no discernible difference whether you print at 400dpi, 300dpi, or 240dpi.
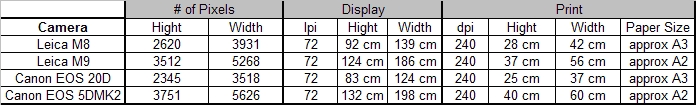
The excerpts displayed on the screen are all approximately 390 pixels wide; the entire M8 image is then about 7 times wider, and the 5DMK2 image is almost 10 times as wide.


At a distance of 30cm, you can survey a print the size of A4 within your field of view without letting your gaze wander. A larger A3 print needs to be viewed from a greater distance to keep it within your field of vision. If you get closer, you'll only see a cropped portion. This eventually turns into what is known as 'Pixel Peeping.' For an A3 print, you wouldn't need to use the full resolution since the angular resolution of the eye hasn't changed. This is also why a poster print from a 10-megapixel camera can still look good; nobody will be examining the poster from 30cm away (unless they want to scrutinize absolute sharpness). As indicated in the table above, all four cameras have a resolution that is more than sufficient for 'normal' applications.
When viewed on the screen at 100% magnification, the shots take on enormous dimensions. It's crucial to keep this in mind when zooming in to 100%. If you later only print on A4 or display the image on the screen, you need a much smaller resolution.
The Used Lenses
I had the following lenses available (the year corresponds to the lens's market introduction):
Canon System:
- EF17-40mmf4-USM-L, 475g, 2003
- EF24-105mmf4-USM-IS-L, 670g, 2005
- EF70-200mmf4-USM-L, 705g, 1999
- EF50mmf1.8 (I), 190g, 1987
- Sigma 105mmf2.8 EX Macro, 450g, 1998
Leica System:
- Summicron 35mmf2.0-ASPH, 255g, 1996
- Summicron 50mmf2.0 (V), 240g, 1994
- Tele-Elmarit 90mmf2.8 (II), 225g, 1973

As you can see, the Canon lenses are relatively more recent, except for the EF50mmf1.8, likely one of the first EF lenses ever. The EF system was introduced in 1987, the same year as the 50mm lens. All Canon lenses weigh over 500g, with the 50mm being an exception at 190g. The Sigma, weighing 450g, is about twice as heavy as the Leica Elmarit with similar optical characteristics. However, the successor lens (the 90mmf2.8 Elmarit) with better optical properties weighs 395g, which is again significantly heavier.
The other Leica lenses also hover around 250g, making them lightweight compared to the Canon zooms. It's worth noting that a Canon 50mm with similar optical characteristics (but not nearly as good mechanical quality) can also be built very lightly. A better Canon 50mm, the EF50mmf1.4, weighs only 290g, comparable to the Leica lenses; however, these lenses are larger, and weight can only be kept low using plastics. The EF lenses also need to accommodate the autofocus motor and aperture drive.
Overall, weight does play a role: Generally, optically superior lenses will weigh slightly more (this is, of course, a highly simplified statement!).
Standard Lens: 50mm Prime
- Canon EF50mmf1.8 (I)
- Leica Summicron 50mmf2.0 (V)

Let's start with a standard lens, an older (1987) Canon 50mm, f/1.8, to serve as the baseline. 50mm lenses can be designed with relatively low effort and achieve very high optical quality. The first example shows the Canon lens, or a crop (always displayed 1:1 here, meaning one screen pixel corresponds to one sensor pixel), once at nearly wide-open aperture f/2.0 and then stopped down to f/4.0, both in the center of the image.


The difference is striking! The better a lens is corrected, the better its performance at wide-open aperture. However, most lenses improve by stopping down due to suboptimal construction. The pictured lens is a good example. At f/4.0, the imaging performance is very good.
For comparison, a 'comparable' lens from the Leica stable, a 50mm Summicron 2.0 (V). I wrote 'comparable' in quotation marks because the Leica lens is priced 5...10 times higher than the Canon lens!


The difference between aperture f/2.0 and f/4.0 is marginal; the second image even appears slightly less sharp. While it's nice to see that stopping down improves the quality of the Canon lens, it also indicates that the lens was suboptimally corrected. A very good lens will show minimal optical performance improvement when stopped down. The old rule of stopping down two apertures or using f/8 for optimal image results is obsolete for truly good lenses.
By the way, the exposure times are slightly different because the Leica has the lowest ISO value of 160, while the Canon was set to ISO 200, making it a third of a stop more sensitive. Similarly, the exposure times for the Canon are a third of a stop shorter. This, of course, has no effect on sharpness.
Now, let's take a look at the image edges. Lenses mostly use spherical lenses, i.e., lenses with a spherical surface. The spherical shape is simply the shape obtained when grinding a lens. Unfortunately, the spherical shape is not optimal for a lens, which is why highly corrected lenses use aspherical lenses (both compared 50mm lenses here have no aspherical lenses). In any case, the suboptimal surface has an impact on the quality of a lens in the edge zones. When stopping down, these edge zones are precisely masked, allowing a lens to gain quality. If a lens already shows poor quality in the center, like the Canon 50mm/1.8, one should not expect too much at the edges:


Here too, stopping down helps. However, the fact that sharpness does not necessarily decrease dramatically towards the edge is once again demonstrated by the Summicron:


But why is the left image brighter than the slightly stopped down one? This is due to vignetting, the darkening towards the edge. This effect can also be improved by stopping down. However, vignetting, like chromatic aberration and geometric distortions, can be easily digitally corrected with a profile, as mentioned earlier. Some cameras do this while the image is being recorded. If not, appropriate measures can be taken later during image editing, as discussed in the article Objektivkodierung.
To further illustrate the comparison of the two lenses, a second example from the image edge is shown below:




In the Canon lineup, there are, of course, better 50mm lenses, such as the 50mm/f1.4. However, I don't own it, so I couldn't compare it. Mechanically, it won't match the Leica lens, but optically, it seems to be excellent.
As the example has shown, 50mm lenses are indeed very good, but there are significant differences. When investing in a prime lens, it's often for the imaging performance or the aperture, and one should not consider the cheapest solution. The superior EF50mmf1.4 from Canon costs around 300 euros used and has an aperture of 1.4, making it a better choice than the 1.8 version. However, it weighs 100g more. The EF50mmf1.8 is available for under 100 euros. For comparison, I acquired the Summicron 50mm used for 1050 euros, but it was practically in new condition. The Summicron is considered one of the best 50mm lenses in its class (f2.0).
Light Telephoto Lenses: 90mm and 105mm
- Sigma 105mmf2.8 EX Macro
- Leica Tele-Elmarit 90mmf2.8 (II)

Let's now compare light telephoto focal lengths, approximately double the focal length of a standard lens. In the Canon system, these are typically macro lenses. Macro lenses are usually well-corrected and, therefore, exhibit excellent characteristics even when used at longer distances. I have my only third-party lens for the Canon system here, a Sigma Macro 105mm, which I inherited from analog times. However, due to its optical performance, I never saw a reason to replace it. In the Leica system, the equivalent focal length is 90mm. Here, the choice is a bit challenging because there's a relatively heavy (by Leica standards!) Summicron with about 500g, or a slightly lighter Elmarit with an aperture of 2.8 weighing 395g, or a much lighter Tele-Elmarit with 225g. I opted for the latter some time ago because it seemed more important to always have a lens with me than to leave a superb piece at home because it's too heavy and large. However, the Tele-Elmarit is over 30 years old (the design dates back to around 1973, almost 40 years old), and unfortunately, you can see that in its optical characteristics. But let's look at the image examples. First, the Sigma:




The Tele-Elmarit is significantly less detailed at wide apertures compared to the Sigma, and even at f4.0, it doesn't reach the same level but improves significantly. However, considering its 30-year-old design, it's not bad at all!
But what about the edges? Again, starting with the Sigma:


Stopping down doesn't improve sharpness; instead, there's a slight degradation, and the vignetting effect becomes more pronounced.


The differences between Leica and Sigma are much smaller here. At f4.0, the Leica is even slightly sharper. Apparently, the sharpness performance of the Leica lens is more uniform across the image frame, showing less noticeable sharpness drop towards the edges.
Zoom Lenses
So far, only prime lenses have been discussed. While these lenses are known for their excellent optical properties, zoom lenses are becoming more prevalent. Technically, they should be called varifocal lenses since the term "zoom" is also used in filmmaking for approaching an object with a fixed focal length. However, "vario" did not catch on, so we stick with "zoom," even though the term is somewhat misleading. A standard zoom lens for the Canon system is the 24-105mmf4L-IS, also offered as the standard lens for the high-resolution Canon 5D Mark II. As we'll see, this is a suboptimal choice. Leica, on the other hand, does not have zoom lenses because the viewfinder can only represent discrete focal lengths. The only comparable option would be a tri-lens, combining three discrete focal lengths.
Since I started using Leica, I have once again realized the importance of using prime lenses. Here, one learns to consciously use a specific focal length and use it accordingly. With a zoom lens, there is a constant temptation to simply turn the zoom until the framing is right. However, while turning the zoom, the perspective changes, and one should have thought beforehand whether to capture the scene with a wide-angle or telephoto lens. But let's not digress and focus on the optical properties.
Normal Zoom: 24-105mm
At 40 and 50mm
Let's first compare at 50mm. First, the EF24-105mmUSM-IS-L, then the EF50mm1.8, and finally, the Summicron 50mmf2.0, all lenses at the edge at f4.0, the maximum aperture of the 24-105mm:



Unfortunately, the zoom lens here fails to impress at all. Even the perceived average Canon 50mm1.8 beats it significantly (at f4.0), and the Summicron is in a league of its own. Considering the weight and size ratios, one might question why one would carry the zoom lens at all, given that it has one stop less light sensitivity.
Just to show that this is not an isolated case, here's a snippet from another shot, again with the same lens sequence, all shots at f4.0 at the edge:



We can also compare the zoom at 40mm with the 17-40mm, all images at f4 at the edge, first the 24-105mm, then the 17-40mm:




As you can see, the two lenses don't differ much; the resolution in both cases is not particularly impressive, but for a zoom lens, it's quite okay.
At 90 and 105mm
Now let's look at other focal lengths, for example, between 90 and 105mm: Canon EF24-105f4.0-USM-IS-L, Sigma 105mmf2.8 EX Macro, Canon EF70-200mmf4.0-USM-L, Leica Tele-Elmarit 90mmf2.8, all at f4.0, center:




Edge:




Firstly, there is joy because the 24-105mm at 105mm is significantly better than at 50mm. Of course, the Sigma is still sharper. However, even the 70-200mmf4-USM-L zoom is slightly better than the Sigma (at f4, at f2.8, the Sigma was a bit sharper), and thus significantly better than the 24-105mm. And when comparing it with the 90mm Tele-Elmarit, one might almost believe that it is impossible to build a zoom that surpasses a 30-year-old prime lens (the 70-200f4 proves the opposite).
Already here, it becomes evident that a prime lens usually demonstrates much better performance than a comparable zoom lens (the EF70-200mmf4.0 at 100mm is, as mentioned, an exception), and yet it is often one or two stops faster, with less weight. It is, of course, clear that a zoom lens carries multiple focal lengths, so you would need several prime lenses to cover a similar range, rendering the weight discussion somewhat absurd. But that is not the only argument for or against zoom/prime lenses. As mentioned earlier, photographic behavior changes completely with prime lenses. One learns to handle each focal length consciously and thinks in advance about whether to use a wide-angle or telephoto lens for a particular subject, positioning oneself accordingly, instead of simply turning the zoom ring. And here the circle closes: Zooming, as mentioned in filmmaking, is changing the distance to the subject with a fixed focal length. Thus, prime lens users are "zoomers" who physically approach (zoom in) or move away (zoom out) from the subject. The "varios" twist the focal length lever.
At 35mm
Let's take a look at the 24-105mm in the wide-angle range. At 35mm, I have an equivalent Leica focal length, and the EF17-40mm also falls into this range. First, the center:



And now the edge:



The excerpts from the center of the image already suggest that the 17-40mm lens is ahead of the 24-105mm, a fact confirmed at the edge, especially note the branches in front of the swimming pool. While the 17-40mm is a 2.35x zoom (the shortest focal length is 2.35 times shorter than the longest focal length), the 24-105mm lens is a 4.375x zoom, so the focal length range is almost twice as large. However, the designs cannot be easily compared, as one is a wide-angle zoom and the other is a normal zoom. Nevertheless, an increased focal length range will always have a negative impact on the imaging performance of the lens. For example, the 70-200mm zoom is a 2.86x zoom, covering a similar range as the 17-40mm zoom.
The 35mm ASPH from Leica stands out from the zoom lenses, with the difference being particularly noticeable at the edge.
Now, of course, the question arises: How much do zoom lenses benefit from stopping down? Here is a comparison of the 24-105mm lens at 35mm focal length at the edge:


And subsequently, the 17-40mm lens, also at 35mm focal length and at the edge:


The gain in sharpness becomes very apparent. Here, the old rule 'at aperture 8 best optical performance' seems to hold true. However, at this point, two stops of light have already been lost, and one must expose four times longer.
Conclusion
In this relatively simple lens comparison, we were able to confirm and also qualify some rules of thumb:
- Prime lenses show significantly better optical imaging performance compared to comparable zoom lenses.
- Prime lenses, with less weight, have an aperture 1-2 stops wider.
- Not all 50mm lenses exhibit good optical characteristics at full aperture.
- Very well-corrected lenses show only marginal gains in optical imaging performance when stopped down, while poorly corrected lenses benefit significantly: Rule of thumb, stop down by two stops.
- The larger the focal length range of a zoom lens with comparable design (size/weight/price), the worse its imaging performance.
- The more elaborate a lens correction, the heavier it becomes.
Links on the Topic
Rangefinder Cameras and the M8
Lens Coding